Thermodynamic Database
PanPhaseDiagram is for phase diagram and thermodynamic property calculation. Thermodynamic database is the prerequisite to fulfill such calculations. A thermodynamic database represents a set of self-consistent Gibbs-energy functions with optimized thermodynamic-model parameters for all the phases in a system. The advantage of CALPHAD method is that the separately-measured phase diagrams and thermodynamic properties can be represented by a unique “thermodynamic description” of the materials system in question. More importantly, on the basis of the known descriptions of the constituent lower-order systems, the thermodynamic description for a higher-order system can be obtained via an extrapolation method [1989Cho]. This description enables us to calculate phase diagrams and thermodynamic properties of multi-component systems that are experimentally unavailable.
In the following, thermodynamic models used to describe the disordered phase, ordered intermetallic phase, and stoichiometric phase are presented. The equations are given for a binary system, and they can be extrapolated to a multi-component system using geometric models [1975Mug, 1989Cho].
The Gibbs energy of a binary disordered solution phase can be written as:
|
|
where the first, second and third terms on the right hand of the equation represent, respectively, the reference states, the entropy of ideal mixing, and the excess Gibbs energy of mixing. Here is the mole fraction of a component
,
is the Gibbs energy of a pure component
, with
structure, R is the gas constant,
is the absolute temperature,
is the interaction coefficient in the polynomial series of the power
. When
, it is a regular solution model, and when
and
, it is a sub-regular solution model. Eq. 1 can be interpolated into a multi-component system using geometric models, such as the Muggianu model [1975Mug].
For a multi-component solution phase with c components, the following equation is used:
|
|
All excess contributions originating from all the binary interactions or ternary interactions are:
|
|
|
|
where
|
|
For ternary systems, xi + xj +xk = 1 and δijk = 0. But for a quaternary or higher system, δijk ≠ 0. In a ternary system, if all the three L parameters are identical,
|
|
Then
|
|
In Pandat, if only one ternary interaction parameter , is provided, Pandat will treat three ternary interaction parameters as the symmetrical case, i.e. all three parameters have the same value. Users can refer to [2005Jan] for more information about ternary interaction parameters.
An ordered intermetallic phase is described by a variety of sublattice models, such as the compound energy formalism [1979Ans, 1988Ans] and the bond energy model [1992Oat, 1995Che]. In these models, the Gibbs energy is a function of the sublattice species concentrations and temperature. The molar Gibbs energy of a binary intermetallic phase, described by a two-sublattice compound energy formalism, , can be written as:
|
|
where and
are the species concentrations of a component,
, in the first and second sublattices, respectively. The first term on the right hand of the equation represents the reference state with the mechanical mixture of the stable or hypothetical compounds:
,
,
, and
.
is the Gibbs energy of the stoichiometric compound,
, with a
structure. The value of
can be obtained experimentally if
is a stable compound; or it can be obtained by ab initio calculation if
is a hypothetical compound. Sometimes,
are treated as model parameters to be obtained by optimization using the experimental data related to this phase. The second term is the ideal mixing Gibbs energy, which corresponds to the random mixing of species on the first and second sublattices. The last three terms are the excess Gibbs energies of mixing. The
parameters in these terms are model parameters whose values are obtained using the experimental phase equilibrium data and thermodynamic property data. These parameters can be temperature dependent. In this equation, a comma is used to separate species in the same sublattice, whilst a colon is used to separate species belonging to different sublattices. The compound energy formalism can be applied to phases in a multi-component system by considering the interactions from all the constituent binaries. Additional ternary and higher-order interaction terms may also be added to the excess Gibbs energy term.
For a reciprocal system with two sublattices, sometimes the interactions among the two species on each sublattice are considered, . The interaction parameter is expressed as [2007Luk]
|
|
Pandat treats the term as the interaction on the first sublattice and the term
as the interaction on the second sublattice.Pandat does not use the alphabetical order of species.
The Gibbs energy of a binary stoichiometric compound ,
, is described as a function of temperature only:
|
|
where is the mole fraction of component
, and
represents the Gibbs energy of component
with
structure,
, which is a function of temperature, represents the Gibbs energy of formation of the stoichiometric compound. If
is a linear function of temperature:
|
|
Then, and
are the enthalpy and entropy of formation of the stoichiometric compound, respectively. Eq. 11 can be readily extended to a multi-component stoichiometric compound phase.
The strategy of building a multi-component thermodynamic database starts with deriving the Gibbs energy of each phase in the constituent binaries. There are constituent binaries in an n-component alloy system, where
|
|
(12) |
To develop a reliable database, the Gibbs energies of these binaries must be developed in a self-consistent manner and be compatible with each other. Three binaries form a ternary, and a preliminary thermodynamic description can be obtained by combining the three constituent binaries using geometric models, such as the Muggianu model [1975Mug]. In some cases, a ternary database developed in this way can describe a ternary system fairly well, while in most cases, ternary interaction parameters are necessary to better describe the ternary system. If a new phase appears in the ternary, which is not in any of the constituent binaries, a thermodynamic model is selected for this ternary phase, and its model parameters are optimized using the experimental information for this ternary phase. There are a total of ternary systems in an n-component system. After thermodynamic descriptions of all
ternaries for an n-component system are established, the model parameters are simply used to describe quaternary and higher-order systems using an extrapolation approach. High-order interaction parameters are usually not necessary because although interactions between binary components are strong, in ternary systems they become weaker, and in higher-ordered systems they become negligibly weak [1997Kat, 2004Cha]. It is worth noting that the term, "thermodynamic database" or simply "database", is usually used in the industrial community instead of "thermodynamic description", particularly for multi-component systems.
At CompuTherm, multi-component databases have been developed for a variety of alloys, such as for Al-alloys, Co-alloys, Cu-alloys, Fe-alloys, Mg-alloys, Mo-alloys, Nb-alloys, Ni-alloys, Ti-alloys, TiAl-alloys, high entropy alloys, and solder alloys. Information for these databases is available in the Databases Manuals from CompuTherm LLC.
Note: The treatment of element sequence in interaction parameters, such as the term in Eq. 3, differs slightly between Pandat and Thermo-Calc. These differences depend on whether the interaction order v is even or odd:
For even values of v , the sequence of elements i and j has no impact because:
=
For odd values of v , the element sequence affects the sign due to the relationship:
=
Consequently, the sign of the interaction parameter must be adjusted accordingly when reversing the element order.
Pandat strictly adheres to the element sequence defined in the TDB file. For example:
G(Hcp,A,B:A;1) =
G(Hcp,B,A:A;1) = and
G(Hcp,A,B:A;1) = -G(Hcp,B,A:A;1)
On the other hand, Thermo-Calc automatically orders elements alphabetically for interaction parameters, irrespective of their sequence defined in the TDB file. i.e.:
G(Hcp,B,A:A;1) = G(Hcp,A,B:A;1) =
To align Pandat with Thermo-Calc’s alphabetical ordering convention, add the following comment in your TDB file
$ software Thermo-Calc
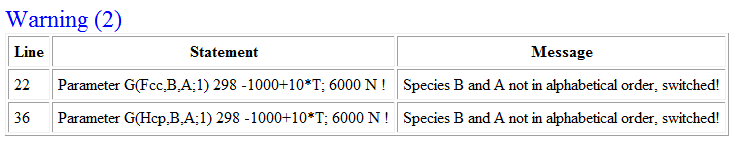
Pandat will then automatically reorder interaction parameters alphabetically and display a warning message confirming the adjustment.
[1975Mug] Y.M. Muggianu et al., “Enthalpies of formation of liquid alloys bismuth-gallium-tin at 723K - choice of an analytical representation of integral and partial thermodynamic functions of mixing for this ternary-system”, J. Chim. Phys., 72 (1975): 83-88.
[1979Ans] I. Ansara, “Comparison of methods for thermodynamic calculation of phase diagrams”, Int. Metal Rev., 24 (1979): 20-53.
[1988Ans] I. Ansara et al., “Thermodynamic modeling of ordered phases in the Ni-Al system”, Acta Metall., 36 (1988): 977-982.
[1989Cho] K.C. Chou et al., “A study of Ternary Geometrical Models”, Ber. Bunsenges. Phys. Chem., 93 (1989): 735-741.
[1992Oat] W.A. Oates et al., “The bond-energy model for ordering in a phase with sites of different coordination numbers”, Calphad, 16 (1992): 73-78.
[1995Che] S.L. Chen et al., “A generalized quasi-chemical model for ordered multicomponent, multi-sublattice intermetallic compounds with anti-structure defects”, Intermetallics, 3 (1995): 233-242.
[1997Kat] U.R. Kattner, “The thermodynamic modeling of multicomponent phase equilibria”, JOM, 49 (1997): 14-15.
[2004Cha] Y.A. Chang et al., “Phase Diagram Calculation: Past, Present and Future”, Prog. Mater. Sci., 49 (2004): 313-345.
[2005Jan] A. Janz, R. Schmid-Fetzer, Impact of ternary parameters, Calphad, 29 (2005) 37-39.
[2007Luk] H. Lukas et al., “Computational Thermodynamics: The Calphad Method”, Cambridge University Press, 2007.